The Geometric Mean Theory of Executive Leadership

Posted on by

As a reader of this blog you may be familiar with the arithmetic mean of a sample:

(1)   \begin{eqnarray*} x_a = \frac{1}{n} \sum_{i=1}^n{ x_i } \end{eqnarray*}

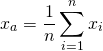
You may also be familiar with the geometric mean of a sample:

(2)   \begin{eqnarray*} x_g = \Big( \prod_{i=1}^n{ x_i } \Big)^{1/n} \end{eqnarray*}

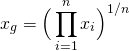
Suppose your sample consists of values 4.0, 4.0, 3.0, and 2.0.   The arithmetic mean is 3.25 and the geometric mean is 3.13.  Pretty similar.   Ah, but suppose your sample consists of values 4.0, 4.0, 3.0, and 0.   The arithmetic mean is 2.75 and the geometric mean is 0.  A single zero has a modest effect on the arithmetic mean but with the geometric mean all it takes is one zero in the sample and the result is zero.  It doesn’t matter how large the other values in the sample are, so long as your sample contains a zero then the geometric mean is zero.  Zero.  Nada.  Zip.

I believe that the geometric mean is a better measure of overall C-suite value than the arithmetic mean.  Why so?  Because the sample size (C-suite population) is small a single zero isn’t easily diluted.  Because all members of the sample wield significant power a single zero has devastating impact on overall value.  In contrast, I believe that the arithmetic mean is a better measure of overall middle management value – larger sample size and limited power of individuals limits the impact of a single zero on the overall quantity.